|
Surds |
|
|
|
To use the resources of this chapter you must first register |
|
|
CONTENTS |
|
|
ITEM |
|
TYPE |
|
NUMBER |
|
| Manipulation of surds |
|
Workout |
|
43 slides |
|
| Surds |
|
Library |
|
16 questions |
|
|
|
Once you have registered, you can work through the slides one by one.
The workout comprises a series of sides that guide you systematically through the topic concept by concept, skill by skill. The slides may be used with or without the support of a tutor. The methodology is based on problem-solving that advances in logical succession by concept and difficulty. The student is presented with a problem or series of questions, and the next slide presents the fully-worked solution. To use the material you must sign-in or create an account.
blacksacademy.net comprises a complete course in mathematics with resources that are comprehensive.
|
|
|
|
SAMPLE FROM THE WORKOUT |
| Showing American English version |
SLIDE 1 - QUESTION 1 |
 |
|
SLIDE 2 - SOLUTION |
 |
|
|
SAMPLE FROM THE LIBRARY |
| Showing American English version |
QUESTION [difficulty 0.1] |
 |
|
SOLUTION |
 |
|
|
DEPENDENCIES |
|
|
|
|
CONCEPTS |
|
|
|
|
LEV. |
|
|
Exact and approximate roots
|
|
722.1 |
|
|
Surd
|
|
722.3 |
|
|
Root terms that are not surds
|
|
722.4 |
|
|
Simplifying surds by extracting square numbers
|
|
722.5 |
|
|
Addition of surds
|
|
723.0 |
|
|
Expand and simplify with surds
|
|
723.2 |
|
|
Binomial products with surds
|
|
723.4 |
|
|
Squares with surds
|
|
723.6 |
|
|
Difference of two squares with surds
|
|
723.8 |
|
|
Rationalising the denominator
|
|
724.0 |
|
|
Conjugate
|
|
724.3 |
|
|
Rationalising by the conjugate
|
|
724.4 |
|
|
Coefficient problem on rationalising a surd
|
|
725.0 |
|
|
Simplifying algebraic fractions with surd denominator
|
|
725.4 |
|
|
Quadratic formula solution with exact surd
|
|
725.7 |
|
|
Algebraic puzzles with surds
|
|
725.8 |
|
|
|
|
RAW CONTENT OF THE WORKOUT |
|
| To make use of this chapter, please first register. Then you can work through the slides one by one. |
|
| What is provided here is the raw text of the workout. Most of the information is contained in the image files, which are not included with this text. The text may appear deceptively short. (The content overall of blacksacademy.net is vast.) Any in-line questions appear as a question mark [?]. This text is provided only as an indication of the overall quantity of material contained in the chapter. To use the material you must sign-in or create an account. |
|
| * |
|
|
Surds
SLIDE 1
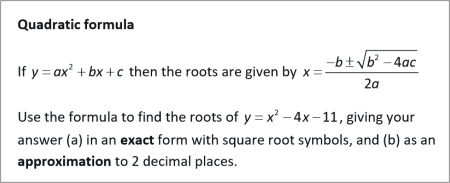
Quadratic formula
If then the roots are given by
Use the formula to find the roots of , giving your answer (a) in an exact form with square root symbols, and (b) as an approximation to 2 decimal places.
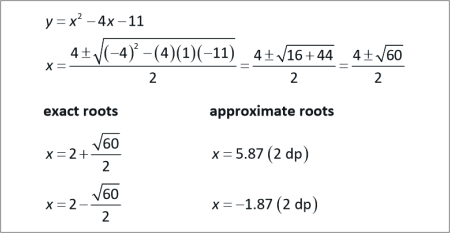
SLIDE 2
exact roots approximate roots
SLIDE 3
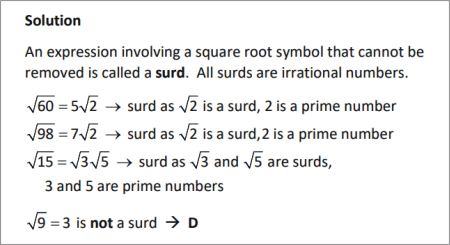
Surds
exact roots approximate roots
An expression involving a square root symbol that cannot be removed is called a surd. All surds are therefore irrational numbers.
In the is a surd.
? Give an example of a surd that is also an irrational number.
? Give an example of a square root that is not an irrational number.
? Find the prime factorisation of 60.
? Is a rational or an irrational number?
SLIDE 4
? The square root of any prime number is an irrational number. Any surd involving a prime number is irrational. For example, , ,
? Give an example of a square root that is not an irrational number.
The square root of any square number is not an irrational number. One might say that it is a rational number masquerading as an irrational one. We unmask it by taking the square root.
?
?
Although 4 is a factor of 60, and , is an irrational number, because it has non-square prime numbers among its factors.
SLIDE 5
Removing square numbers
Numbers appearing under a root symbol should be simplified by taking any square numbers outside the root symbol.
The square roots of prime numbers cannot be simplified.
Note
means 2 times , following the usual rule that when things are placed together unambiguously, it means the product.
SLIDE 6
Simplify by removing square numbers outside the root symbol where possible.
? ? ?
? ? ?
SLIDE 7
?
?
?
?
?
?
SLIDE 8
Example
Simplify in the same way
? ? ?
? ? ?
SLIDE 9
?
?
?
?
?
?
SLIDE 10
Example
Exercise
Collect like terms
?
?
?
SLIDE 11
?
?
?
SLIDE 12
Example
Note
Exercise
Expand and simplify
?
?
?
SLIDE 13
?
?
?
SLIDE 14
Example
Exercise
Expand and collect
?
?
?
SLIDE 15
?
?
?
SLIDE 16
Expand and collect
?
?
SLIDE 17
?
?
SLIDE 18
Example
Exercise
Expand and collect
?
?
?
?
SLIDE 19
?
?
?
?
SLIDE 20
Rationalising the denominator
We wish to remove a surd from the bottom (denominator) of a fraction.
Since , the way to remove from the bottom of a fraction is to multiply it by . To preserve the equation, we multiply both the top (numerator) and bottom (denominator) of the fraction by , as .
SLIDE 21
Rationalise the denominator of each of the following.
? ?
? ?
SLIDE 22
?
?
?
?
SLIDE 23
Conjugate
The conjugate of is because
The surd is eliminated when multiplied by the conjugate.
Example
By multiplying both numerator and denominator of by the conjugate of , rationalise the denominator.
Solution
SLIDE 24
By multiplying both numerator and denominator by the conjugate of the denominator, rationalise the denominator.
? ?
SLIDE 25
?
?
SLIDE 26
By multiplying both numerator and denominator by the conjugate of the denominator, rationalise the denominator.
? ?
SLIDE 27
?
?
SLIDE 28
By multiplying both numerator and denominator by the conjugate of the denominator, rationalise the denominator.
? ?
SLIDE 29
?
?
SLIDE 30
Simplify giving your answer in the form where a and b are integers.
SLIDE 31
SLIDE 32
Write in the form where a and b are integers.
SLIDE 33
SLIDE 34
Example
Exercise
Simplify
? ?
? ?
SLIDE 35
?
?
?
?
SLIDE 36
Solve each of the following, giving your answers as an exact surd in their simplest form
?
?
SLIDE 37
?
?
SLIDE 38
Solve giving your answers in the form , where a and b are integers.
SLIDE 39
Solve giving your answers in the form , where a and b are integers.
Solution
SLIDE 40
If , which of the following is a possible value of ?
A
B
C
D
E
SLIDE 41
If , which of the following is a possible value of ?
Solution
The question asks us to solve for , not for x. Because of this, the question is a “trick” question. In fact, we may directly solve for as follows.
The answer is E
If you solve for x, you obtain
Then , and the same answer.
Note, option C, gives a zero in the denominator of , and is not defined for this value.
SLIDE 42
ERROR IN QUESTION CORRECTED BELOW. CHECK THE SLIDE.
If , find the possible values of
SLIDE 43
|
|