|
Finding roots |
|
|
|
To use the resources of this chapter you must first register |
|
|
CONTENTS |
|
|
ITEM |
|
TYPE |
|
NUMBER |
|
| Roots and iterative methods |
|
Workout |
|
28 slides |
|
| Roots and iterative processes |
|
Library |
|
9 questions |
|
|
|
Once you have registered, you can work through the slides one by one.
The workout comprises a series of sides that guide you systematically through the topic concept by concept, skill by skill. The slides may be used with or without the support of a tutor. The methodology is based on problem-solving that advances in logical succession by concept and difficulty. The student is presented with a problem or series of questions, and the next slide presents the fully-worked solution. To use the material you must sign-in or create an account.
blacksacademy.net comprises a complete course in mathematics with resources that are comprehensive.
|
|
|
|
SAMPLE FROM THE WORKOUT |
| Showing American English version |
SLIDE 1 - QUESTION 1 |
 |
|
SLIDE 2 - QUESTION 1 |
 |
|
|
SAMPLE FROM THE LIBRARY |
| Showing American English version |
QUESTION [difficulty 0.5] |
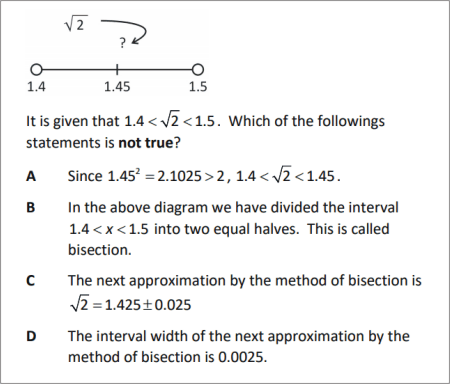 |
|
SOLUTION |
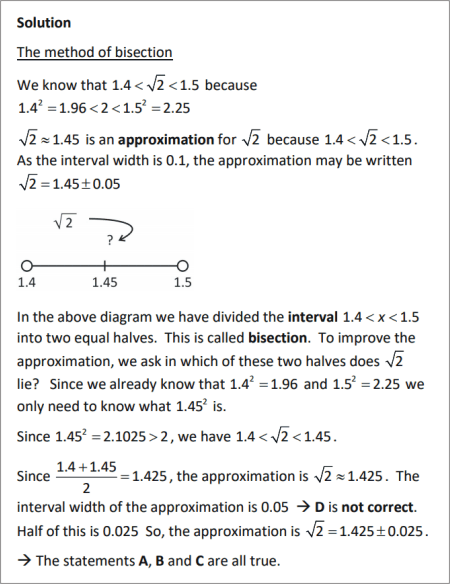 |
|
|
DEPENDENCIES |
|
|
|
|
CONCEPTS |
|
|
|
|
LEV. |
|
|
Consolidation of method of bisection
|
|
762.1 |
|
|
Consolidaton of method of trial and improvement
|
|
762.6 |
|
|
Speed of convergence
|
|
762.6 |
|
|
Consolidation of exact and inexact solutions
|
|
762.9 |
|
|
Locating a root within an interval
|
|
763.1 |
|
|
Systematic search for a root
|
|
763.2 |
|
|
Iterative methods
|
|
764.1 |
|
|
Fixed point iterative method (implicit) by rearrangement
|
|
764.1 |
|
|
Adding subscripts n, n+1, iterative formula
|
|
764.1 |
|
|
Numerical solution
|
|
764.1 |
|
|
|
|
RAW CONTENT OF THE WORKOUT |
|
| To make use of this chapter, please first register. Then you can work through the slides one by one. |
|
| What is provided here is the raw text of the workout. Most of the information is contained in the image files, which are not included with this text. The text may appear deceptively short. (The content overall of blacksacademy.net is vast.) Any in-line questions appear as a question mark [?]. This text is provided only as an indication of the overall quantity of material contained in the chapter. To use the material you must sign-in or create an account. |
|
| * |
|
|
ROOTS AND ITERATIVE METHODS
SLIDE 1
Revision - the method of bisection
We know that because
is an approximation for because . As the interval width is 0.1, the approximation may be written
In the above diagram we have divided the interval into two equal halves. This is called bisection. To improve the approximation, we ask in which of these two halves does lie? Since we already know that and we only need to know what is.
Since , we have .
Since , the approximation is . The interval width of the approximation is 0.5. Half of this is 0.025
So, the approximation is
SLIDE 2
Given , iterate the method of bisection twice to find an approximation for to an accuracy of .
SLIDE 3
because
The interval width of the approximation is 0.25. Half of this is 0.0125. The approximation is
because
SLIDE 4
Given , use the method of bisection to find an approximation for to an accuracy of .
SLIDE 5
Given , use the method of bisection to find an approximation for to an accuracy of .
Solution
SLIDE 6
Revision - trial and improvement
Trial and improvement is an iterative method that uses educated guesswork to improve the speed of convergence on a value.
We use the information given in the process to choose an interval that is more likely to give quicker convergence.
Example
Given , use trial and improvement to find an approximation for to 3 decimal places
SLIDE 7
Given , use trial and improvement to find an approximation for to 3 decimal places.
SLIDE 8
Given , use trial and improvement to find an approximation for to 3 decimal places.
Solution
SLIDE 9
? Use the quadratic formula to find the roots of the quadratic function . Give your answers as exact values in the form , where a and b are integers.
? Find and . Find an approximation to to 2 decimal places.
? Sketch the graph of for . Mark the points and onto your sketch. Mark the root onto your sketch.
? Referring to your sketch, what happens to the sign of as it crosses the x-axis at the root in the interval .
? What kind of number is ? Is it possible to find an exact decimal for ?
SLIDE 10
?
?
?
? The graph of as it crosses the x-axis at the root in the interval changes from negative to positive.
? is an irrational number. It is not possible to find an exact decimal for . It has an infinite, not-repeating decimal expansion.
SLIDE 11
Locating a root
Suppose is a root of the function .
Then there is a change of sign in the value of in the region close to .
has a sign change in the interval ? has a root in that interval.
*
Alternative wording
If is negative (positive) at , and positive (negative) at , then has a root in the interval .
SLIDE 12
Example
This function has a negative root. Find an interval of width 1 which contains the negative root.
Solution
We search systematically for the root by substituting negative integer values for x in .
SLIDE 13
This function has a negative root. Find an interval of width 1 which contains the negative root.
SLIDE 14
This function has a negative root. Find an interval of width 1 which contains the negative root.
Solution
SLIDE 15
Expand and collect the above and hence show that
can be written as a product of a linear and a quadratic factor.
SLIDE 16
Hence, is the product of a linear and a quadratic factor.
SLIDE 17
This function has a root in the interval . Use the method of trial and improvement to find to an accuracy of 2 decimal places.
SLIDE 18
has a root in the interval . Use the method of trial and improvement to find to an accuracy of 2 decimal places.
Solution
SLIDE 19
has a root in the interval . By trial and improvement, we have obtained .
? Let be the two roots of , where . Use the quadratic formula to find , giving your answers in surd form, and hence factorise in term of completely .
? Confirm that the negative root of is to 2 decimal places and state as an exact surd.
SLIDE 20
?
where
? We confirm that
SLIDE 21
To iterate means to repeat a process. An iterative method in mathematics is a method that uses a value from a previous calculation to make a further improvement in a numerical solution to a problem.
Example
To find the negative root of
We start with a rearrangement of the equation where x is the subject. At this level, this rearrangement is given.
We confirm that is a rearrangement of
We add subscripts to the left-hand side and right-hand side of the rearrangement respectively.
This is an iterative formula.
SLIDE 22
Example continued
Use the iterative formula , starting with to find the negative root, , of . Give the results of your iterations to 5 decimal places, and halt your iterations when your final solution is accurate to 3 decimal places.
Solution
Since to 3 decimal places, we stop at the 7th iteration.
SLIDE 23
? Show that is a rearrangement of .
? Using the iterative formula , and starting with a value of find the negative root, , of to 1 decimal place.
? Use the quadratic formula to solve . State the negative root, , of in an exact form using surds.
? What is the relationship between ?
SLIDE 24
?
?
?
? is 1 decimal place approximation to .
SLIDE 25
? By expanding , show that
has a quadratic factor.
? Find the negative root of this quadratic factor, giving your exact answer in the form , where a and b are integers.
? Show that is a rearrangement of .
? Using the iterative formula and starting with , find an approximation, , to the negative root of . Give your answer to 2 decimal places.
? Comment on the relationship of , and state the negative root of .
SLIDE 26
?
Therefore, is a quadratic factor of .
?
?
?
? is the negative root of .
SLIDE 27
x is a number such that
? Find two consecutive whole numbers between which x must lie.
? Use the method of trial and improvement to find x correct to 3 significant figures.
? . Find constants A, B and C such that . State the root of .
SLIDE 28
?
We compute for integer values of x and look for a sign change. (Alternative, compare with 20.)
?
?
The root of is
|
|