|
Intersection of lines and curves |
|
|
|
To use the resources of this chapter you must first register |
|
|
CONTENTS |
|
|
|
|
|
Once you have registered, you can work through the slides one by one.
The workout comprises a series of sides that guide you systematically through the topic concept by concept, skill by skill. The slides may be used with or without the support of a tutor. The methodology is based on problem-solving that advances in logical succession by concept and difficulty. The student is presented with a problem or series of questions, and the next slide presents the fully-worked solution. To use the material you must sign-in or create an account.
blacksacademy.net comprises a complete course in mathematics with resources that are comprehensive.
|
|
|
|
SAMPLE FROM THE WORKOUT |
| Showing American English version |
SLIDE 1 - QUESTION 1 |
 |
|
SLIDE 2 - SOLUTION |
 |
|
|
SAMPLE FROM THE LIBRARY |
| Showing American English version |
QUESTION [difficulty 0.5] |
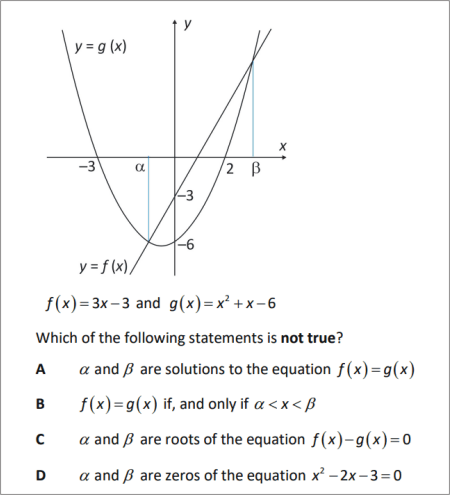 |
|
SOLUTION |
 |
|
|
DEPENDENCIES |
|
|
|
|
CONCEPTS |
|
|
|
|
LEV. |
|
|
Intersection of line and parabola
|
|
766.1 |
|
|
Intersection of line and hyperbola
|
|
766.5 |
|
|
System of three equations (curves)
|
|
766.7 |
|
|
Simulataneous equations - one linear one non-linear
|
|
766.9 |
|
|
Formation of non-linear simultaneous equations
|
|
767.3 |
|
|
Discarding a solution
|
|
767.6 |
|
|
Number of solutions to f(x) = 0 by sketching
|
|
767.9 |
|
|
f(x) = g(x) --> f(x) - g(x) = 0
|
|
768.1 |
|
|
|
|
RAW CONTENT OF THE WORKOUT |
|
| To make use of this chapter, please first register. Then you can work through the slides one by one. |
|
| What is provided here is the raw text of the workout. Most of the information is contained in the image files, which are not included with this text. The text may appear deceptively short. (The content overall of blacksacademy.net is vast.) Any in-line questions appear as a question mark [?]. This text is provided only as an indication of the overall quantity of material contained in the chapter. To use the material you must sign-in or create an account. |
|
| * |
|
|
Intersection of line and curve
SLIDE 1
? On the same diagram, sketch the graphs of and .
? What do the intersections between the line and the parabola you have sketched represent?
? How many solutions are there to ?
SLIDE 2
?
? The intersections represent the solutions to the equation
? There are two intersections. Therefore, there are two solutions to . These are marked at and in the diagram.
SLIDE 3
and
? Find the two solutions and to the equation .
? Find the points of intersection of .
? In terms of what are the solutions to the equation ?
SLIDE 4
and
?
? When
The points of intersection at
? are the solutions to the equation . Hence the are the roots of .
SLIDE 5
? On the same diagram, sketch the graphs of and . What kind of curves are these?
? Solve the equation for x, and find the points of intersection of and .
SLIDE 6
and
? is a straight line, and is a hyperbola.
?
SLIDE 7
The diagram shows the graphs of a system of three equations. How many simultaneous solutions does the system have?
SLIDE 8
The only simultaneous solution to all three equations is the point where all three lines intersect, corresponding to in the diagram. The system has exactly 1 solution.
SLIDE 9
Solve the simultaneous equations
SLIDE 10
SLIDE 11
Solve the simultaneous equations
SLIDE 12
SLIDE 13
The area of a rectangle is 45 square units, and its perimeter is 28 units. Find the dimensions of the rectangle.
SLIDE 14
The area of a rectangle is 45 square units, and its perimeter is 28 units. Find the dimensions of the rectangle.
Solution
Let the length of the width of the rectangle be x and the length y.
area is 45 square units ?
perimeter is 28 units ?
To solve
The dimensions are 5 and 9 units.
SLIDE 15
The diagram shows the plan for a patio, which comprises a rectangle with one side 8 m as shown to which is attached a semi-circular area. If the total area of the patio is find the length and width of the patio.
SLIDE 16
The diagram shows the plan for a patio, which comprises a rectangle with one side 8 m as shown to which is attached a semi-circular area. If the total area of the patio is find the length and width of the patio.
Solution
If the radius of the semi-circular part is r then the breadth of the patio is . The area of the rectangle is and the area of the semi-circle is half the area of a circle, . The total area is 100. Hence, .
The negative solution has no meaning in the context of this problem, so we discard it. .
The breadth is
The length is
SLIDE 17
By equating coefficients find constants A and B such that
SLIDE 18
SLIDE 19
Given that for all x, how many roots does have, and find all of them.
SLIDE 18
Since for all x, has only one root. This root is to be found where its factor , which is at .
SLIDE 20
The figure shows a sketch of the function
? Make a copy of the sketch and add to it a sketch of the function .
? Using the sketch, state the number of solutions to the equation .
? Find the cubic function .
? To what point or points on your sketch does correspond?
SLIDE 21
?
? There is only point of intersection ? has only one solution.
?
? The point to which is the simultaneous solution to at in the figure.
SLIDE 22
and
Given that is a factor of find such that . Find the coordinates of the point of intersection where .
SLIDE 23
and
Given is a factor of , let
|
|