|
Algebraic manipulations |
|
|
|
To use the resources of this chapter you must first register |
|
|
CONTENTS |
|
|
ITEM |
|
TYPE |
|
NUMBER |
|
| Algebra puzzles and manipulations |
|
Workout |
|
62 slides |
|
| Algebraic manipulations |
|
Library |
|
17 questions |
|
|
|
Once you have registered, you can work through the slides one by one.
The workout comprises a series of sides that guide you systematically through the topic concept by concept, skill by skill. The slides may be used with or without the support of a tutor. The methodology is based on problem-solving that advances in logical succession by concept and difficulty. The student is presented with a problem or series of questions, and the next slide presents the fully-worked solution. To use the material you must sign-in or create an account.
blacksacademy.net comprises a complete course in mathematics with resources that are comprehensive.
|
|
|
|
SAMPLE FROM THE WORKOUT |
| Showing American English version |
SLIDE 1 - QUESTION 1 |
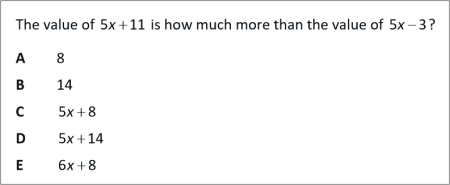 |
|
SLIDE 2 - SOLUTION |
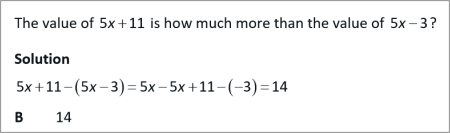 |
|
|
SAMPLE FROM THE LIBRARY |
| Showing American English version |
QUESTION [difficulty 0.1] |
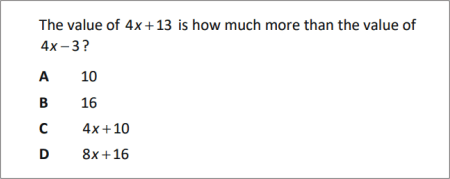 |
|
SOLUTION |
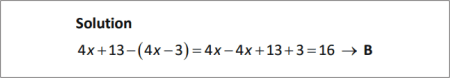 |
|
|
DEPENDENCIES |
|
|
|
|
CONCEPTS |
|
|
|
|
LEV. |
|
|
Simple algebra puzzles
|
|
708.1 |
|
|
Factorisation puzzles
|
|
708.3 |
|
|
Expand and collect puzzles
|
|
708.5 |
|
|
Equating coefficients
|
|
708.9 |
|
|
Puzzles on coefficients
|
|
709.2 |
|
|
Harder difference of two squares
|
|
709.6 |
|
|
Number factorisation problem
|
|
710.0 |
|
|
Harder factorisation
|
|
710.2 |
|
|
Consolidation: singularity
|
|
710.6 |
|
|
Harder singularity problems
|
|
710.7 |
|
|
Blunder of not recognising a singularity
|
|
710.9 |
|
|
The square root with negative solutions
|
|
711.4 |
|
|
Solution set problem
|
|
711.7 |
|
|
Simplifying algebraic fractions by first factorising
|
|
711.9 |
|
|
Factorising simple cubic expressions
|
|
712.1 |
|
|
Quotients of functions
|
|
712.3 |
|
|
Coefficient problem on rational expressions
|
|
712.5 |
|
|
Quartic expression as disguised quadratic
|
|
712.9 |
|
|
Algebraic proof of number property
|
|
713.2 |
|
|
Algebraic proof in recurring decimal expansion
|
|
713.6 |
|
|
Problems on volumes and surface areas
|
|
714.1 |
|
|
|
|
RAW CONTENT OF THE WORKOUT |
|
| To make use of this chapter, please first register. Then you can work through the slides one by one. |
|
| What is provided here is the raw text of the workout. Most of the information is contained in the image files, which are not included with this text. The text may appear deceptively short. (The content overall of blacksacademy.net is vast.) Any in-line questions appear as a question mark [?]. This text is provided only as an indication of the overall quantity of material contained in the chapter. To use the material you must sign-in or create an account. |
|
| * |
|
|
Algebraic manipulations
SLIDE 1
The value of is how much more than the value of ?
A 8
B 14
C
D
E
SLIDE 2
The value of is how much more than the value of ?
Solution
B 14
SLIDE 3
If what is the value of ?
A
B 3
C
D 19
E 192
SLIDE 4
If what is the value of ?
Solution
B 3
SLIDE 5
Which of the following is equivalent to ?
A
B
C
D
E
SLIDE 6
Solution
E
SLIDE 7
Which of the following expressions is equivalent to the one above?
A
B
C
D
SLIDE 8
Solution
A
SLIDE 9
Example
By expanding the left-hand side and equating coefficients, find a, b and c.
Solution
SLIDE 10
By equating coefficients, find a, b and c.
SLIDE 11
SLIDE 12
The sum of and can be written in the form , where a, b and c are constants. What is the value of ?
SLIDE 13
The sum of and can be written in the form , where a, b and c are constants. What is the value of ?
Solution
SLIDE 14
Given , find .
SLIDE 15
SLIDE 16
? Factorise
? Let and . By substituting into your answer to part ?, find .
SLIDE 17
?
? Given and and , then
Note,
SLIDE 18
The expression can be written in the form , where a and b are constants. Find .
SLIDE 19
The expression can be written in the form , where a and b are constants. Find .
Solution
Hence
SLIDE 20
Factorise
?
?
? Find without a calculator
? Find without a calculator
SLIDE 21
Factorise
?
?
?
?
SLIDE 22
Factorise
?
?
?
?
SLIDE 23
?
?
?
?
SLIDE 24
Factorise
?
?
?
?
?
SLIDE 25
?
?
?
?
?
Note. In the last by comparison with , which is the difference of two squares, where and .
SLIDE 26
Singularity
No function is defined where a denominator is equal to zero. For example, the function is undefined when , which is when .
The reason for this is that in algebra it is never permitted to divide by zero. Any value where a function is undefined is called a singularity.
SLIDE 27
State the values for which the following functions are undefined
?
?
?
?
?
SLIDE 28
?
?
?
?
?
SLIDE 29
What is invalid (incorrect, wrong) about the following?
What should be the correct solution to the equation?
SLIDE 30
Strictly, we should write provided that .
where is short for “x is not equal to 1”.
Incorrect Correct
We cannot have a solution because is undefined at . The correct and only answer is .
SLIDE 31
Which of the following are all the solutions to the above?
A
B
C
D
SLIDE 32
Solution
C only
SLIDE 33
? Substitute and into , taking only the positive square root in each case.
Consider the following
? Substitute into each line of the above, taking and state whether the line is true or false.
? Substitute into each line of the above, allowing and state whether the line is true or false.
? State the solutions of .
SLIDE 34
? Substituting and into and taking only the positive square root
? Substituting into each line
? Substituting into each line
? In fact, both and are solutions to because can be negative.
SLIDE 35
Someone argues as follows
On substituting into we obtain
Hence, cannot be a solution to .
Explain what is wrong with this argument and show that is a solution to
SLIDE 36
The error in the argument is that . So, we have
To show that is a solution to
SLIDE 37
Which of the following is the solution set to ?
A
B
C
D
E
SLIDE 38
However, is not a solution to the original equation.
Substitution of gives and . The reason why is not a solution is that in we cannot have a negative value under the root symbol. Thus . Hence the negative is not a solution. The answer is B, only.
SLIDE 39
Simplify
? ?
? ?
SLIDE 40
?
?
?
?
SLIDE 41
Factorize
?
?
?
SLIDE 42
?
?
?
SLIDE 43
Find and simplify
SLIDE 44
SLIDE 45
Find a, b and c for each of the following
?
?
SLIDE 46
?
?
SLIDE 47
Find a, b and c for each of the following
?
?
SLIDE 48
?
?
SLIDE 49
Example
By substituting into solve
Solution
SLIDE 50
By substituting or otherwise solve
?
?
SLIDE 51
?
?
SLIDE 52
If n is an integer, prove that the sum of and is a square number.
SLIDE 53
If n is an integer, prove that the sum of and is a square number.
Solution
SLIDE 54
Let n be an integer. Prove that
is the product of three consecutive number.
SLIDE 55
SLIDE 56
Example
Prove algebraically that
Solution
Let , then
In general
If is an infinitely recurring decimal expansion,
then .
If is an infinitely recurring decimal expansion,
then .
If is an infinitely recurring decimal expansion,
then .
Likewise, for any recurring decimal expansion.
SLIDE 57
? Find the fraction equivalent to , expressing this fraction in its lowest terms.
? Prove algebraically that is the fraction you found.
SLIDE 58
?
? To prove algebraically that
Proof
Let , then
SLIDE 59
Prove algebraically that
SLIDE 60
Prove algebraically that
Solution
SLIDE 61
A sphere has been cut into four equal quarters. The volume of each quarter is . Find the surface area of each quarter, giving your answer to 3 significant figures.
SLIDE 62
A sphere has been cut into four equal quarters. The volume of each quarter is . Find the surface area of each quarter, giving your answer to 3 significant figures.
Solution
The surface is in three parts. The “outer” part is of the surface of the sphere, and the two “inner” parts are both semicircles passing through the centre of the sphere.
SLIDE 61B
A sphere has been cut into four equal quarters. The volume of each quarter is . Find the surface area of each quarter, giving your answer to 3 significant figures.
SLIDE 62B
A sphere has been cut into four equal quarters. The volume of each quarter is . Find the surface area of each quarter, giving your answer to 3 significant figures.
Solution
The surface is in three parts. The “outer” part is of the surface of the sphere, and the two “inner” parts are both semicircles passing through the centre of the sphere.
|
|